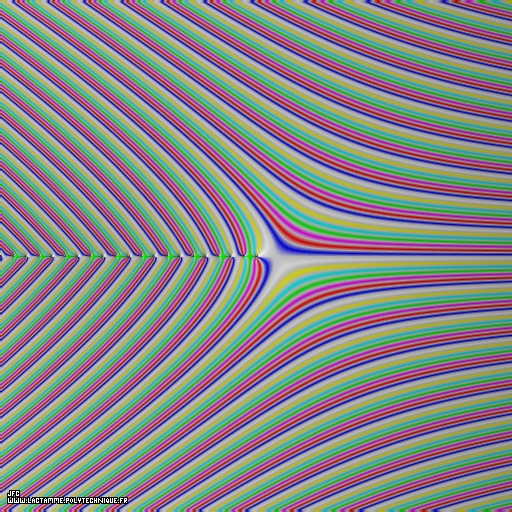
Tridimensional display of the Gamma function inside (-20.0,20.0)x(-20.0,20.0) (bird's-eye view) [Visualisation tridimensionnelle de la fonction Gamma dans (-20.0,20.0)x(-20.0,20.0) (vue aérienne)].

                    Gamma(z) = factorial(z-1)

                                                                     __
                                              1                log(2 ||)
                    log(factorial(z)) = (z + ---)log(z) - z + ------------
                                              2                    2

                                          k=V
                                        _______
                                        \             B
                                         \             2k
                                      +  /      ---------------
                                        /______           2k-1
                                                 2k(2k-1)z
                                          k=1

                                      + epsilon(z,N,V)


                                      factorial(z+n)
                    factorial(z) = --------------------
                                    (z+1)(z+2)...(z+n)

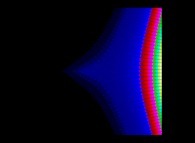• the absolute value of the Real part of Gamma(z),
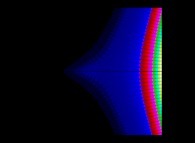• the absolute value of the Imaginary part of Gamma(z),
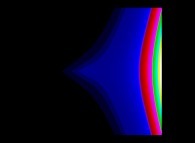• the Modulus of Gamma(z),
• the Phase of Gamma(z).