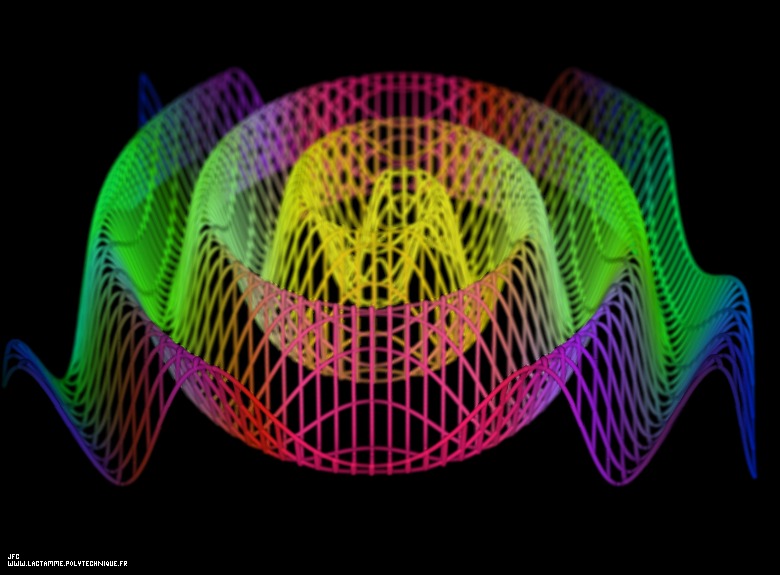
A 'spiraling plane' defined by means of three bidimensional fields with depth of field effect [Un 'plan spiralant' défini à l'aide de trois champs bidimensionnels avec de la profondeur de champ].

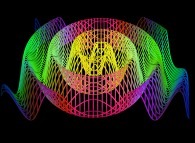See this surface without the depth of field effect.

                    X = F (u,v)
                         x

                    Y = F (u,v)
                         y

                    Z = F (u,v)
                         z

                    u E [U   ,U   ]
                          min  max

                    v E [V   ,V   ]
                          min  max

                       v ^
                         |
                    V    |...... ---------------------------
                     max |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                         |      |+++++++++++++++++++++++++++|
                    V    |...... ---------------------------
                     min |      :                           :
                         |      :                           :
                         O------------------------------------------------->
                                U                           U              u
                                 min                         max

                    X = M (i,j)
                         x

                    Y = M (i,j)
                         y

                    Z = M (i,j)
                         z

                    i = f(u,U   ,U   ,N )
                             min  max  u

                    j = g(v,V   ,V   ,N )
                             min  max  v

• Fx
• Fy
• Fz