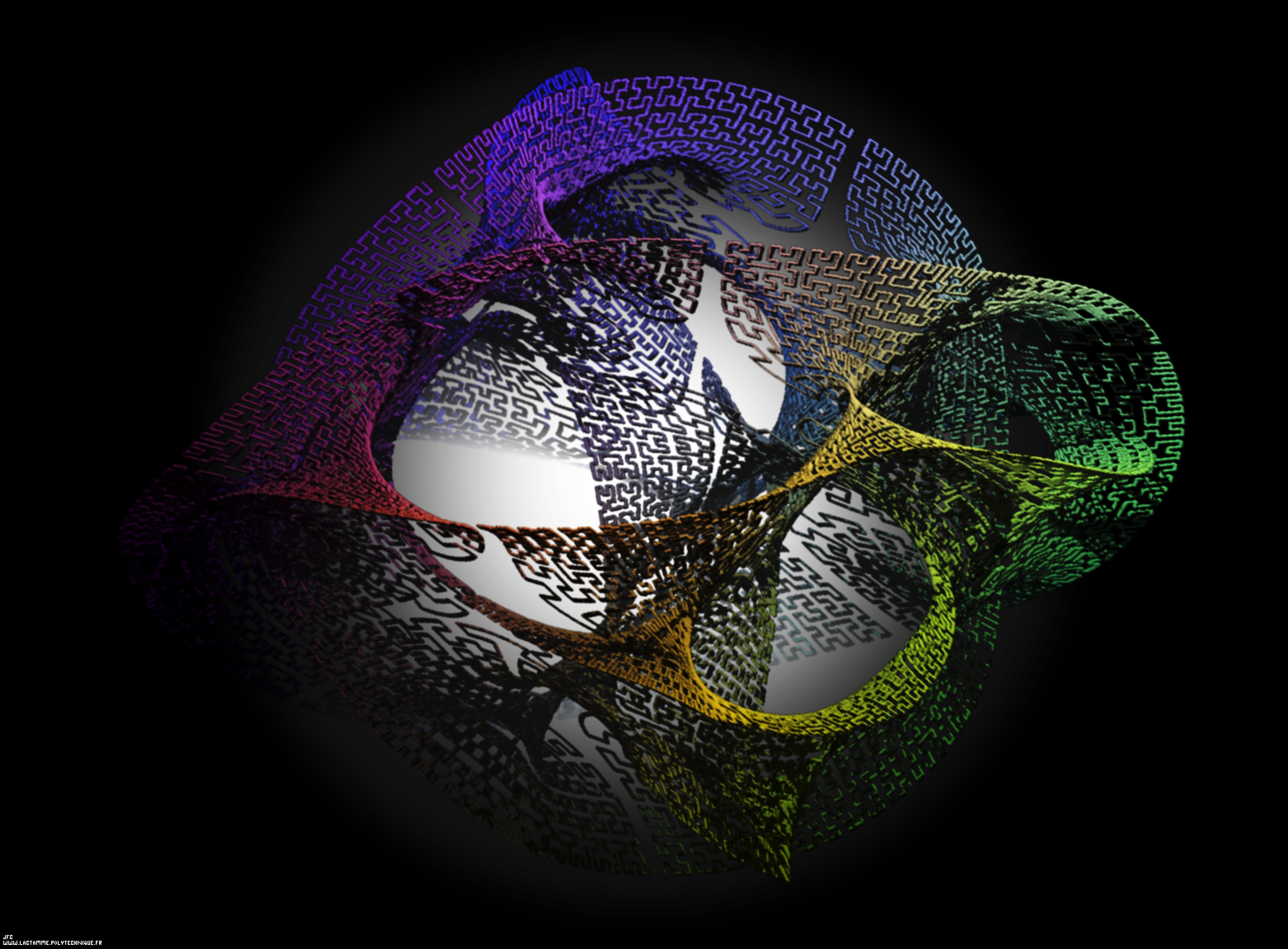
Tridimensional representation of a quadridimensional Calabi-Yau manifold described by means of 5x5 Bidimensional Hilbert Curves -iteration 5- [Représentation tridimensionnelle d'une variété quadridimensionnelle de Calabi-Yau décrite à l'aide de 5x5 courbes de Hilbert bidimensionnelle -itération 5-].

